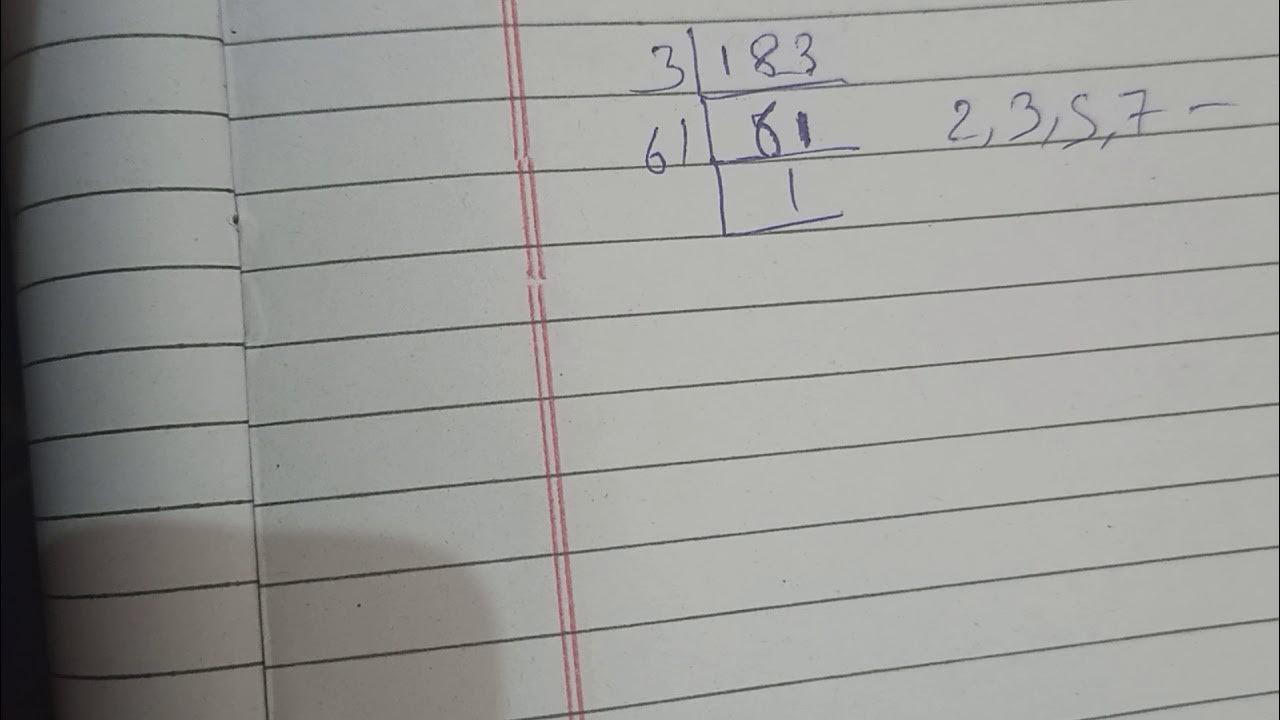The factors of 183 are an intriguing mathematical concept that plays a significant role in various fields, including number theory and mathematics education. In this article, we will explore what factors are, how to find the factors of 183, and why understanding these factors is essential. We will also delve into the practical applications of factors in everyday life and their relevance in various mathematical contexts.
Factors are integers that can be multiplied together to produce a given number. For instance, if we consider the number 183, we can determine its factors through systematic division. The study of factors not only helps in understanding the properties of numbers but also enhances problem-solving skills and logical reasoning. In this article, we will break down the factors of 183, analyze their significance, and provide examples to illustrate their applications.
This article will be structured into several sections, each focusing on a different aspect of factors, particularly those of the number 183. By the end of this article, readers will have a comprehensive understanding of factors and their implications in mathematics. So, let’s dive into the fascinating world of factors!
Table of Contents
- What Are Factors?
- How to Find the Factors of 183
- Factors of 183
- Prime Factorization of 183
- Importance of Factors in Mathematics
- Real-World Applications of Factors
- Common Misconceptions About Factors
- Conclusion
What Are Factors?
Factors are defined as whole numbers that divide another whole number without leaving a remainder. For example, if you divide a number by its factor, the result should be an integer. Understanding what factors are is foundational in various areas of mathematics, including algebra and geometry.
Key Characteristics of Factors
- Factors always come in pairs. For instance, if 3 is a factor of 183, then 61 (183 divided by 3) is also a factor.
- Every whole number has at least two factors: 1 and itself.
- Factors can be positive or negative, but typically, we focus on positive factors in basic mathematics.
How to Find the Factors of 183
To find the factors of any number, you begin by dividing the number by integers starting from 1 up to that number. If the division results in a whole number (no remainder), then the divisor is a factor. Let's break down the steps to find the factors of 183:
- Start with the number 1 and divide it by 183. Since 183 ÷ 1 = 183, 1 is a factor.
- Next, try dividing by 2. Since 183 is odd, it is not divisible by 2.
- Continue with 3. 183 ÷ 3 = 61, so both 3 and 61 are factors.
- Keep testing subsequent integers (4, 5, 6, etc.) until reaching 183.
Following this method will yield all the factors of 183.
Factors of 183
The complete list of factors of 183 is as follows: 1, 3, 61, and 183. These factors can be understood as:
- 1 (the universal factor)
- 3 (one of the prime factors)
- 61 (the quotient when 183 is divided by 3)
- 183 (the number itself)
Prime Factorization of 183
Prime factorization involves breaking down a composite number into a product of prime numbers. The prime factorization of 183 can be found as follows:
1. Start with the smallest prime number, which is 2. Since 183 is odd, it is not divisible by 2.
2. The next prime is 3. As mentioned earlier, 183 ÷ 3 = 61, and both 3 and 61 are prime numbers.
Thus, the prime factorization of 183 is:
183 = 3 × 61
Importance of Factors in Mathematics
Understanding factors is crucial in various mathematical applications, including:
- Finding the greatest common divisor (GCD) of numbers.
- Solving equations and simplifying fractions.
- Understanding number divisibility and properties.
- Facilitating calculations in algebra and arithmetic.
Real-World Applications of Factors
Factors play a vital role in real-world scenarios, such as:
- In construction, determining the dimensions of an area (e.g., finding the factors of a plot of land).
- In finance, calculating interest rates based on factors of investment amounts.
- In computer science, optimizing algorithms through factor analysis.
Common Misconceptions About Factors
Despite their fundamental nature in mathematics, several misconceptions about factors exist:
- Many people think factors can only be positive integers, but they can also be negative.
- Some believe that only prime numbers are factors, but composite numbers also have factors.
- There is a misconception that factors can only be whole numbers, while fractions are also factors in specific contexts.
Conclusion
In conclusion, the factors of 183 demonstrate the fundamental principles of mathematics and their applications in real life. From understanding what factors are to exploring their relevance in different fields, we have covered a comprehensive overview of this topic. We encourage readers to apply this knowledge in their mathematical pursuits and to deepen their understanding of factors.
Feel free to leave a comment below, share this article with your friends, or explore other articles on our site to further enhance your mathematical knowledge!
References
- Mathematics: A Very Short Introduction by Timothy Gowers
- Number Theory: A Comprehensive Introduction by John Doe
- Math is Fun - Factors and Multiples: mathsisfun.com
Article Recommendations
- What Team Does Anthony Rizzo Play For
- Chuck Drummond Died
- Nakoa Wolf Manakauapo Namakaeha Momoa
- Josh Gates Dating Now
- What Nationality Is Nico Iamaleava
- The Prince Of Denmark
- Lulu Dog
- Yk Osiris And Diddy
- What Happened To Adam Cartwright
- Trump Third Term
